Improper integrals I Nathan P ueger 30 September 2011 1 Introduction An improper integral is an integral that is unbounded in one of two ways. Lets take a second and think about how the Comparison Test works.
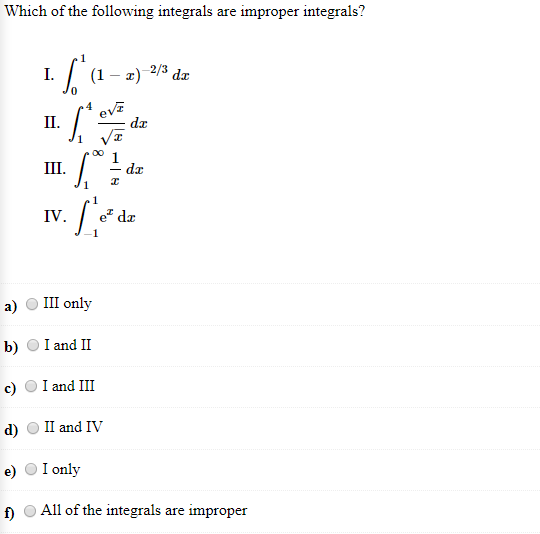
Solved Which Of The Following Integrals Are Improper Chegg Com
Either the domain of integration is in nite or the function approaches in nity in.
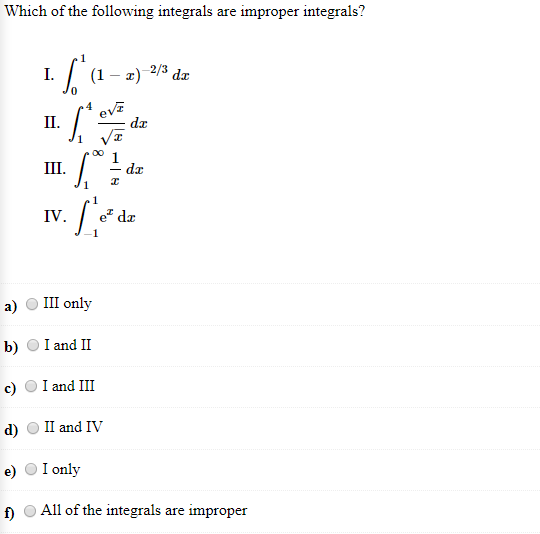
. P X 8 8 3 e 3 x d x lim t t 8 3 e 3 x d x lim t e 3 x t 8 lim t e 3 t e 24 38 10 11. 39 displaystyle 9_0fracdxsqrt9x Answer. B Lets guess that this integral is divergent.
And we know when the resulted limit is finite when the power of x is greater than 1 I mean that x 3 2 the improper integral a converges. Example 1 Determine if the following integral is convergent or divergent. MATH 142 - Improper Integrals Joe Foster Example 2.
That means we need to nd a function smaller than 1e x x that is divergent. However its not that difficult to multiple the sinx to make it larger than 1. One way to think of that integral is by approximating delta by triangular pulse functions D_n for n.
Then determine whether the integral converge or diverge. The value 38 10 11 represents the probability of no accidents in 8. Find the values of alpha for which the improper integral iint_Df_alphaxydxdy exists 7 Proof of connection between improper Riemann Integral and Lebesgue integral.
Each of these integrals has an infinite discontinuity either at an endpoint or at an interior point of the interval. If it is divergent type Diverges or D. 2 cos2x x2 dx 2 cos 2 x x 2 d x.
Divergent if the limit does not exist. B because sinπ 0 so the integrand is undefined at π which is in the interval of integration. If we divide it to 3 parts we have.
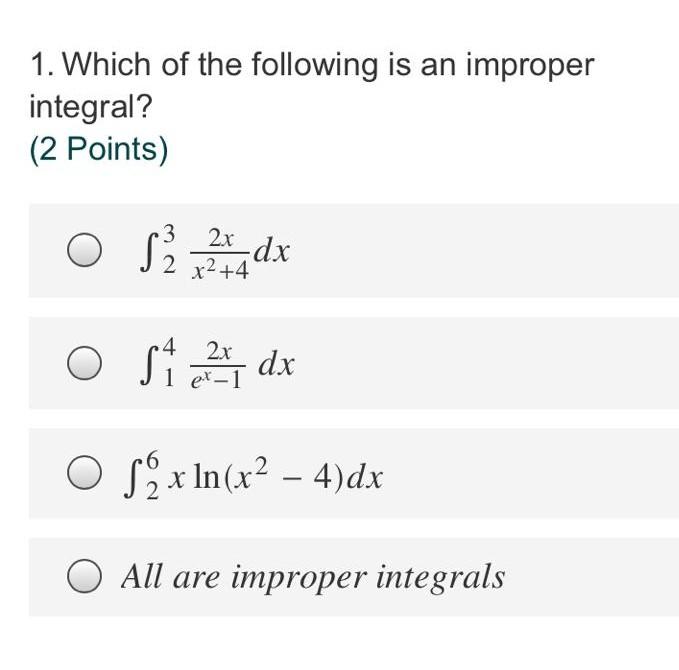
Which of the following is an improper integral. Convergence is good means we can do the integral. First Practice on Improper Integrals February 25 2020 Background Whenever a 1 or b 1or both the integral Z b a fx dx is said to be an improper integral.
Given improper integral 0 e 2 t d t. The improper integral int_-inftyinfty deltaxdx 1 is one of the notable improprieties of the Dirac delta function delta. Lets choose c 0 and write ˆ 1 1x2 dx ˆ0 1 1x2 dx ˆ 0 1 1x2 dx.
TT 2 Points 1 dx 1 20 x2-16 II S. Question 4 1 point Compute the value of the following improper integral. Improper integrals are always handled by taking limits of ordinary integrals.
A Improper because it is an in nite integral called a Type I. Explain and write the improper integral in limits form. 1 point Match the following improper integral with the improper integral below in which you can compare using the Comparison Test.
Clearly this integral converges when 0 a 1 but that doesnt help us because our test integral is less than the original. 1 e x x 1 x Then take the integral. If this integral is convergent then well need to find a larger function that also converges on the same interval.
Which of the following integrals are improper. Transcribed image text. According to part 3 of Definition 1 we can choose any real number c and split this integral into two integrals and then apply parts 1 and 2 to each piece.
Select all that apply 2 In x - 1 dx 1 - O 3 1 dx 2 3x 1 La incx 3x La be 8 sin x dx 1 4x2 o 1 1 dx 2x 1 -. E 2 t 2 a 0. Dx 14- III SO 1216 dx 1 and III III only 1 only II and III.
Which of the following integral is improper. Lets make the top smaller. In exercises 39 - 44 evaluate the improper integrals.
Which of the following integral is improper. Lim x x 3 2 1 x 3 2 x 2 1. Applying limit by the value a tends to.
Calculus questions and answers. If the limit is finite we say the integral converges while if the limit is infinite or does not exist we say the integral diverges. Which of the following is an improper integral.
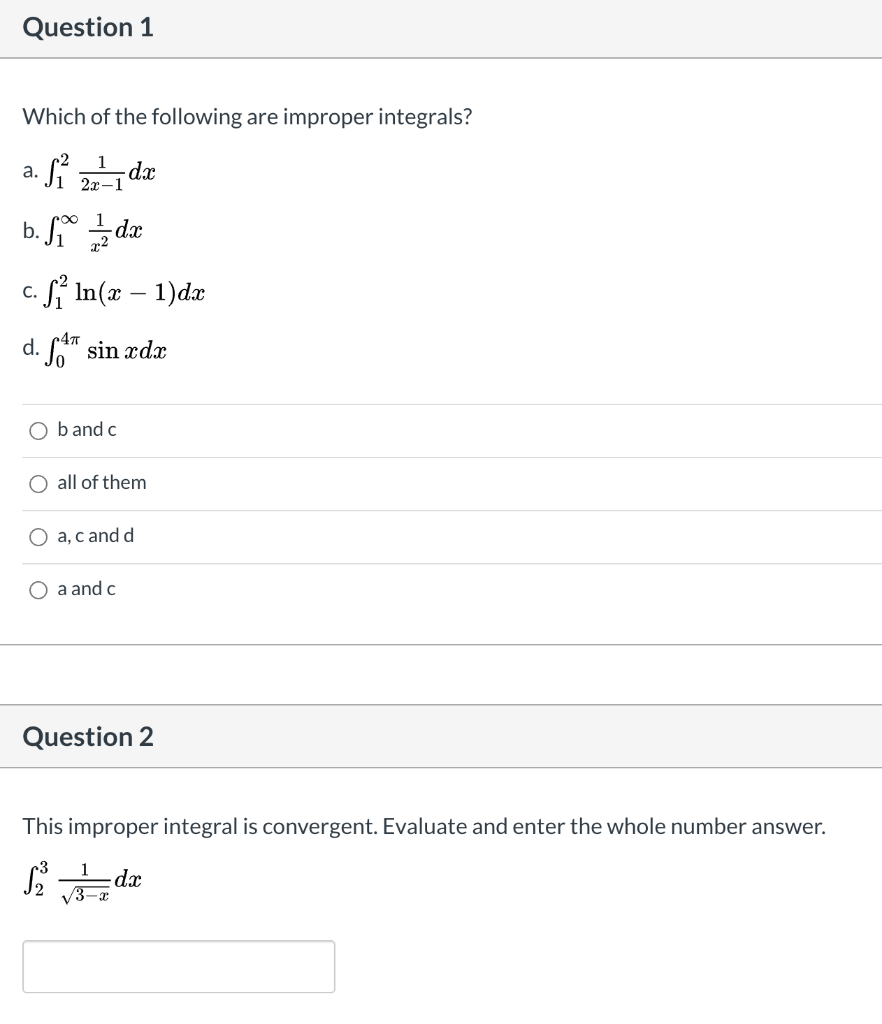
0 5 0 2 2 3 3 5. 0 e 2 t d t lim a 0 a e 2 t d t. Which of the following integrals are improper.
We need to calculate the probability as an improper integral. Experts are tested by Chegg as specialists in their subject area. Improper integrals are integrals you cant immediately solve because of the infinite limits or vertical asymptote in the interval.
To make it smaller we can make the top smaller or the bottom bigger. Explain and write the improper integral in limits form do not evaluate the integral 4 c. TT 2 Points 1 dx 1 20 x2-16 II S.
Either one of its limits are infinity or the integrand that function inside the interval usually represented by fx goes to infinity in the integral. 0 e 2 t d t lim a. The reason you cant solve these integrals without first turning them into a proper integral ie.
0 e 2 t d t 1 2 e 2 1 2 e 2 0 0 e 2 t d t 05. Evaluate ˆ 1 1x2 dx. We say R 1 a fx dxconverges if the limit lim b1 Z b a fx dx exists and we say the integral diverges.
Dx 14- III SO 1216 dx 1 and III III only 1 only II and III II only. If C 3 then it is of order x 1 and the integral diverges. Solved Which of the following integrals are improper.
Which of the following is an improper integral. Integral1_-1 x dx integral2_0 lnx2 dx integral0_-e e2x dx None of these Calculate the area under the graph of fx 1x2 above the x-axis on the interval 1 infinity. Z 1 1 1 x dx lim t1 Z t 1 1 x dx lim t1 lnx t.
Each integral on the previous page is defined as a limit. Consider the first part. The integrand is continuous for x 0 so the only possible problem is the infinite interval.
A is improper because one of the limits of integration is infinite. D because the integrand is undefined at 0 and 0 is in the. Math 1B lecture 11.
I 0 3 C x 2 3 x C x 2 1 3 x 1 d x. We review their content and use your feedback to keep the quality high. If C 3 then the integrand is of the order of x 2 and the integral converges.
Solved 1 Which Of The Following Is An Improper Integral 2 Chegg Com
Solved Question 1 Which Of The Following Are Improper Chegg Com

Solved Which Of The Following Are Improper Integrals Chegg Com
0 Comments